
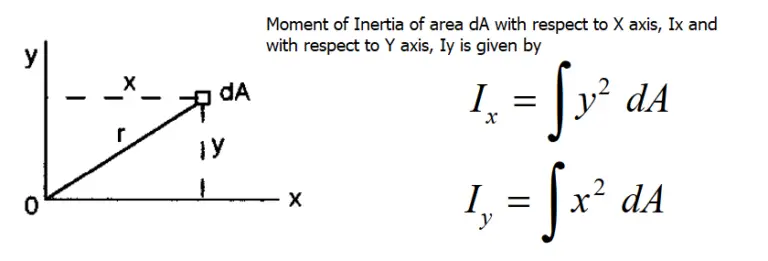
The greater its value, the greater the moment required to provide a given acceleration about a fixed pivot. The mass moment of inertia about a fixed axis is the property of a body that measures the body's resistance to rotational acceleration. The 10 6 factor removes unwanted digits from the value. Moment of inertia is the rotational analogue to mass. It may be +ve, -ve, or zero Product of Inertia of area A w.r.t. A standard method of denoting moment of inertia is to write the values as: number x 10 6 mm 4. Integrating curvatures over beam length, the deflection, at some point along x-axis, should also be reversely proportional to I. Area Moments of Inertia Products of Inertia: for problems involving unsymmetrical cross-sections and in calculation of MI about rotated axes. Therefore, it can be seen from the former equation, that when a certain bending moment M is applied to a beam cross-section, the developed curvature is reversely proportional to the moment of inertia I. Beam curvature κ describes the extent of flexure in the beam and can be expressed in terms of beam deflection w(x) along longitudinal beam axis x, as: \kappa = \frac. Where E is the Young's modulus, a property of the material, and κ the curvature of the beam due to the applied load.

If we take the axis that passes through the base, the moment of inertia of a triangle is given as I bh 3 / 12. The bending moment M applied to a cross-section is related with its moment of inertia with the following equation: The moment of inertia of a triangle having its axis passing through the centroid and parallel to its base is expressed as I bh 3 / 36. The moment of inertia (second moment or area) is used in beam theory to describe the rigidity of a beam against flexure (see beam bending theory). For a uniform solid cuboid, the moment of inertia is taken to be about the vertical axis passing through the cuboid's center of mass and perpendicular to a side. The term second moment of area seems more accurate in this regard. The mass moment of inertia measures the extent to which an object resists rotational acceleration about a particular axis, and is the rotational analog to mass. This is different from the definition usually given in Engineering disciplines (also in this page) as a property of the area of a shape, commonly a cross-section, about the axis. Centroid of a Circle Arear2 A r e a r 2, Ix4r4 I x 4 r 4 Iy4r4 I y 4 r 4, Jz2r4. It is related with the mass distribution of an object (or multiple objects) about an axis. Centroids and Area Moments of Inertia for 2D Shapes Circle. In Physics the term moment of inertia has a different meaning. The dimensions of moment of inertia (second moment of area) are ^4.


 0 kommentar(er)
0 kommentar(er)
